The E-graph extraction problem is NP-complete

In this quick note, I will show that the E-graph extraction problem is NP-complete.

The E-graph extraction problem is defined as follows:

 Input: An E-graph G and a cost function mapping E-nodes
        to positive numbers.
Output: A DAG t represented by G such that t has the
        lowest cost possible.

First, the extraction problem is in NP¹ because it can be reduced to integer linear programming (ILP). Moreover, we show the extraction problem is NP-hard by reducing the minimum set cover problem to it.

The minimum set cover problem is defined as follows (adapted from Wikipedia):

 Input: A set of elements {1, 2, ..., n} (called the universe)
        and a collection S of m sets whose union equals the 
        universe.
Output: The smallest sub-collection of S whose union equals
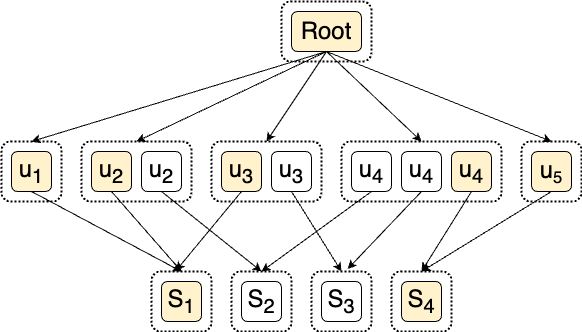
        the universe. 

We show an instance of how this minimum set cover problem can be reduced to E-graph extraction: consider the universe \(U = \{1, 2, 3, 4, 5\}\) and the collection of sets \(S = \{ \{1, 2, 3\}, \{2, 4\}, \{3, 4\}, \{4, 5\} \}\). The smallest subset of \(S\) that covers all of the elements is { {1, 2, 3}, {4, 5} }.

Our construction is as follows:

1. For each \(j\in U\), we create a corresponding E-class \(c_{j}\).
2. For each collection \(S_i\), we create an E-class \(c_{S_i}\) with a singleton E-node \(S_i\).
3. For each \(S_i=\{j_1, \ldots, j_{l_m}\}\), we create a new E-node \(u_{j_k}(c_{S_i})\) in E-class \(c_{j_k}\) for all \(j_k\).
4. We create a root E-class with a special E-node whose children include all \(C_{j_k}\).
5. Every E-node has a uniform cost of 1.²

This will produce the following E-graph for our example.

The intuition behind this construction is that, to extract the root E-class, we have to cover all the elements in the universe, so we need to pick an E-node from each \(c_{j}\). To cover all \(c_{j}\)’s with the smallest cost means picking as fewer \(S_i\) E-nodes as possible, which corresponds to a minimum set cover.

As a side note, the construction here uses function symbols with non-constant arities (i.e., the root E-node). This can be fixed by replacing the root E-node with \(O(n)\) many E-nodes with binary function symbols forming a depth \(O(\log n)\) binary tree, so our reduction only requires unary and binary function symbols.

Update (Aug 29, 2023): Michael Stepp showed the NP-completeness of this problem via a similar reduction to Min-SAT in his thesis.